1. What is an RLC Circuit Calculator?

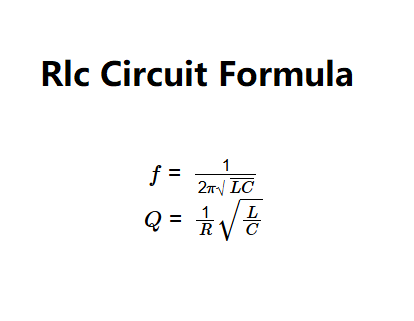
Definition: This calculator computes the resonant frequency (f) and Q-factor (Q) of an RLC circuit, which consists of a resistor (R), inductor (L), and capacitor (C).

Purpose: It is used in electronics to analyze RLC circuits, critical for designing oscillators, filters, and tuned circuits in radio and audio applications.

2. How Does the Calculator Work?

The calculator uses the following equations:

Where:

• \( f \) is the resonant frequency (Hz)
• \( L \) is the inductance (H)
• \( C \) is the capacitance (F)
• \( R \) is the resistance (Ω)
• \( Q \) is the Q-factor (unitless)
• \( \pi \) is approximately 3.14159

Steps:

• Enter capacitance (C), inductance (L), and resistance (R)
• Convert inputs to base units (F, H, Ω)
• Calculate \( f \) and \( Q \) using the formulas
• Display \( f \) in Hz/kHz/MHz and \( Q \) as a number or ∞ if R = 0

3. Importance of RLC Circuit Calculation

The resonant frequency determines the oscillation frequency, while the Q-factor indicates the circuit's selectivity and efficiency. A higher Q means sharper resonance and longer-lasting oscillations.

4. Using the Calculator

Examples:

• For \( C = 2 \, \text{pF} \), \( L = 1 \, \text{µH} \), \( R = 1 \, \text{kΩ} \):
  • \( f = \frac{1}{2\pi \sqrt{1 \times 10^{-6} \cdot 2 \times 10^{-12}}} = 112.53754 \, \text{MHz} \)
  • \( Q = \frac{1}{1000} \sqrt{\frac{1 \times 10^{-6}}{2 \times 10^{-12}}} = 0.70711 \)
• For \( C = 100 \, \text{nF} \), \( L = 5 \, \text{µH} \), \( R = 10 \, \text{Ω} \):
  • \( f = \frac{1}{2\pi \sqrt{5 \times 10^{-6} \cdot 100 \times 10^{-9}}} = 71.17846 \, \text{kHz} \)
  • \( Q = \frac{1}{10} \sqrt{\frac{5 \times 10^{-6}}{100 \times 10^{-9}}} = 0.70711 \)

5. Frequently Asked Questions (FAQ)

Q: What is an RLC circuit?
A: An RLC circuit is a resonant circuit with a resistor, inductor, and capacitor, used in applications requiring specific frequency responses.

Q: What does a low Q-factor mean?
A: A Q-factor below 0.5 indicates heavy damping, causing oscillations to die out quickly. Higher Q values are preferred for sharper resonance.

RLC Circuit Calculator© - All Rights Reserved 2025